

Slinky and Shear Slinky are graphical tools for investigating solutions of initial value problems for ordinary differential equations. The codes were developed for a particular systems of ordinary differential equations that model the equilibria of elastic rods, and the rest of this page will describe the packages in that context. However simple modifications would allow other systems to be investigated too. The basic idea of the packages is to interactively send initial values to an ODE IVP solver (in this case the implementation of an Adams Pece method as described in the book Computer Solution of Ordinary Differential Equations: The Initial Value Problem by L.F. Shampine and M.K. Gordon) from within a graphics package and to visualize various `projections' of the associated trajectories.
Slinky and Shear Slinky were developed by Kathleen A. Hoffman and Randy C. Paffenroth at the Institute for Physical Science and Technology's Numerical Analysis Lab at the University of Maryland, College Park. The programs were developed within the AVS graphical environment.
The motivation for the development of slinky and shear slinky is an interest in using an elastic rod model to approximate the supercoiling of DNA molecules. Members of the Numerical Analysis Laboratory are involved in a number of related projects including dynamic models, but slinky deals with statics. Within static models, boundary value problems also arise but slinky deals with initial value problems only. Slinky models an unshearable inextensible rod, and shear slinky models an extensible shearable rod.
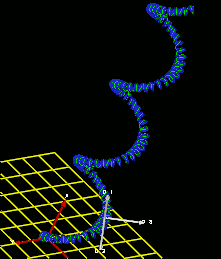
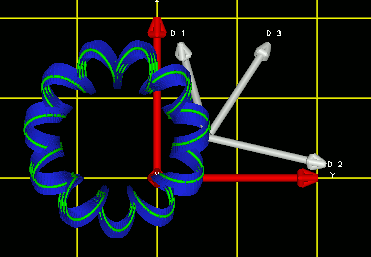
The configuration space in the elastic rod model used in Slinky comprises a curve with an associated frame that evolves with the arclength of the curve. The `moving' frame consists of a set of three directors which give information about the orientation of the cross section of the rod. One of the directors that is normal to the curve is used to construct a ribbon to help visualize the twist in the rod. Slinky colors the curve, or center line, green, the ribbon blue, and the director axes white. One of Slinky's features it that it can animate the directors moving along the arclength of the curve.
The equilibrium equations solved by Slinky are a set of first order ordinary differential equations that are of Hamiltonian form. In these equations of `motion' evolution is with respect to arc length as the independent variable.
The current version of Slinky only allows uniform rods. The coefficients in the system of ODEs are therefore constant. The constants are related to the unstressed configuration of the rod and shape and orientation of the cross section of the rod. The moments of inertia and physical constants such as the Young's modulus and Poisson's ratio, combine to form the stiffness parameters labelled K1, K2, and K3, respectively. The parameters labelled `u-hat' described the unstressed configuration of the rod. The actual input in the program are the radius and pitch of a helix. Slinky then transforms these parameters to the appropriate unstressed bending and twisting parameters. These coeffients form part of the parameter space. The particular choice of initial conditions completes the parameter space. For instance, the configuration variable's initial condition is always fixed at the origin. The initial orientation of the directors is controlled by two angles input by the user. The initial forces also need to be specified. For this particular application, we have chosen to embed the forces so that only one component, namely, the first component is non-zero. The user also has control over the length of the rod that is displayed.
The parameter space breaks down into two categories, the integrable case and the non-integrable case. In the integrable case, the two moments of inertia of the cross sections of the rod are equal. This is, K1=K2. The unstressed configuration of the rod must be straight as well. Concomitantly, the parameters describing the radius and pitch of the unstressed helix are zero. The configuration space of this case can be described as a helix upon a helix. In fact for this case the IVP can be solved by quadrature (see Shi and Hearst, The Kirchhoff Elastic Rod, the Nonlinear Schrodinger Equation, and DNA Supercoiling, Journal of Chemical Physics, vol.101, number 6, 1994, for example) The centerline of the rod is confined within two cylinders. Viewing the rod along the long axis of the cylinder, it appears that the solutions are wrapped on a torus.
The non-integrable case does not place any restrictions on the parameters. The resulting solutions do not have the same structure as with the integrable case, but some periodicity can be seen. Slinky is designed to be able to compute all cases.
Shear Slinky is an analogous program for shearable, extensible elastic rods. Shearing describes lateral movement of the cross section across arc length. The shearability parameters used by Shear Slinky measure the shear in the principal axes of the cross section. The extensibility parameter measures how much the arc-length of the rod will either extend or contract under a force or torque. Thus Shear Slinky has three extra parameters in its parameter space. The unshearable, inextensible rod, which Slinky deals with, always has the third director tangent to the rod. This is not the case for the shearable extensible case. Thus in Shear Slinky we have added a magenta tangent vector , whose magnitude is a measure of the shear and extensibility of the rod. Special thanks go to Brian Michalowski for this feature.